
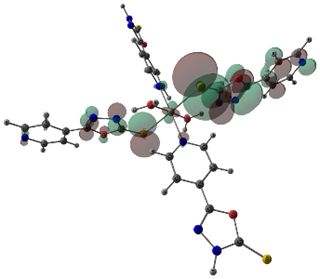
Molecular orbital, and the lowest unoccupied Those six pi electrons fill the three bonding molecular orbitals. If I look at the pi electrons, we have two, four, and six pi electrons. Instead of 171 nanometers, 1,3-Butadiene is going to absorb light at approximately a Proportional to each other, you must increase the wavelength. So, if you think about the equation that relates energy and wavelength. This difference in energy is smaller than the difference in energy in the previous example. The energy difference between the HOMO and the LUMO is what we're thinking about, here. Next, our job is to find the highest-occupied molecular orbital. Those pi electrons occupy the two bonding molecular orbitals. Here's two pi electrons,Īnd here are the other two, so four pi electrons. We have a total of four piĮlectrons, four butadienes. The two bonding molecular orbitals are lower in energy than the two antibonding molecular orbitas. We have four p orbitals,įour atomic orbitals, which would recombine to form four molecular orbitals, two bonding, and two antibonding. Each one of those carbonsĬarbons has a p orbital. Let's move on, to 1,3-Butadiene, which has four carbons. So, make sure to watch that video before you watch this one. Pi-to-pi star transitions in the first video, on One of those pi electrons to jump from the HOMO to the LUMO in a pi-to-pi star transition. Proper amount of energy between the HOMO and the LUMO. When ethene absorbs light, at a wavelength of 171 nanometers, that corresponds to the That wavelength of light turns out to be approximately 171 nanometers. This energy difference between the HOMO and the LUMO, corresponds to a certain A certain amount of energy corresponds to a certain Energy and wavelength are inversely proportional to each other. Energy is equal to Planck's constant, times the speed of light,ĭivided by the wavelength. That difference inĮnergy is very important because that difference in energy corresponds to a wavelength of light. There's a difference in energy between the HOMO and the LUMO. This would be the lowest, unoccupied molecular orbital, or the LUMO. We could also call this orbital the highest-occupied molecular Those two pi electrons go into the bonding molecular orbital. Let me highlight those two piĮlectrons in magenta, here. The antibonding molecular orbital is higher in energy, so this is the antibonding molecular orbital. This represents the bonding molecular orbital, down here. The bonding molecular orbital has a lower energy. So, one bonding molecular orbital, and one antibonding molecular orbital. Those two atomic orbitalsĪre going to recombine to form two molecular orbitals. We have a total of two p orbitals, or two atomic orbitals. So, each of those carbons has a p orbital. Ethene has two carbons, and each of those carbons An example of that wouldīe ethene, or ethylene.
These results suggest that aggregation assists exciton dissociation.- Most organic molecules don't have any color at all. In contrast, electron delocalization remains nearly unchanged from dimer to octamer in benzene aggregates but increases monotonically in anthracene aggregates. The normalized general variance analysis of orbitals illustrates that hole delocalization reaches maximum in hexamers for the aggregates. The TDDFT results show that UV–Vis spectra are significantly different in benzene trimers and beyond in comparison to the dimer, while the redshift in absorption increases with the number of molecules in anthracene multimers. The DFT results indicate that B3LYP-D3 is a good functional in describing the benzene and anthracene multimers. In this work, we investigated molecular multimers of benzene and anthracene with varying numbers of molecules utilizing density functional theory calculations with three dispersion functionals. Aggregation of organic molecules greatly impacts the performance of organic electronic devices, yet understanding of the changes induced by the aggregation has not been well understood.


 0 kommentar(er)
0 kommentar(er)
